Interviews are mapmaking sessions where the interviewer and interviewee endeavor to understand each other better. In order to do that, they must ask strong, probing questions. Unfortunately, many interviews fall short of their intended purpose.
But it doesn’t have to be this way.
In the book Zero to One: Notes on Startups or How to Build the Future, Peter Thiel shares an interview question he dubs “The Contrarian Question.” It goes like this:
What important truth do very few people agree with you on?
There’s a lot to unpack with this question.
Reason #1 for Asking the Contrarian Question
Thiel asks this question because it’s both intellectually and psychologically difficult to answer.
This question sounds easy because it’s straightforward. Actually, it’s very hard to answer. It’s intellectually difficult because the knowledge that everyone is taught in school is by definition agreed upon. And it’s psychologically difficult because anyone trying to answer must say something she knows to be unpopular. Brilliant thinking is rare, but courage is in even shorter supply than genius.
Difficult questions like the contrarian question are important to ask because they force the person on the receiving side to really think and show some originality. Can they effectively deviate from the crowd? And if so, do they have the courage to show it?
Because this question is so hard—and unorthodox—Thiel gets a lot of bad answers. Many include:
- Our educational system is broken and needs to be fixed.
- America is exceptional.
- There is no God.
The reason these are poor types of answers is that they are common. Examples one and two might be true, but they are commonly held beliefs for many. Example three is simply taking a stance on a well-known debate; many people agree with you. A good answer addresses the part of the question asking about very few people agreeing with you.
Thiel formulates a good answer as the following:
“Most people believe in x, but the truth is the opposite of x.”
Reason #2 for Asking the Contrarian Question
Thiel asks this question because it provides different perspectives about the world around us and what the future might hold.
No one can predict the future exactly, but we know two things: it’s going to be different, and it must be rooted in today’s world. Most answers to the contrarian question are different ways of seeing the present; good answers are as close as we can come to looking into the future.
Thinking about the future is important because that’s where we are going. And we have some influence over what that future might look like. Ultimately, we hope that the future is better than the present and that we progress as time passes.
That progress comes in two forms: horizontal and vertical.
Horizontal or extensive progress means copying things that work—going from 1 to n. Horizontal progress is easy to imagine because we already know what it looks like. Vertical or intensive progress means doing new things—going from 0 to 1. Vertical progress is harder to imagine because it requires doing something nobody else has ever done. If you take one typewriter and build 100, you have made horizontal progress. If you have a typewriter and build a word processor, you have made vertical process.
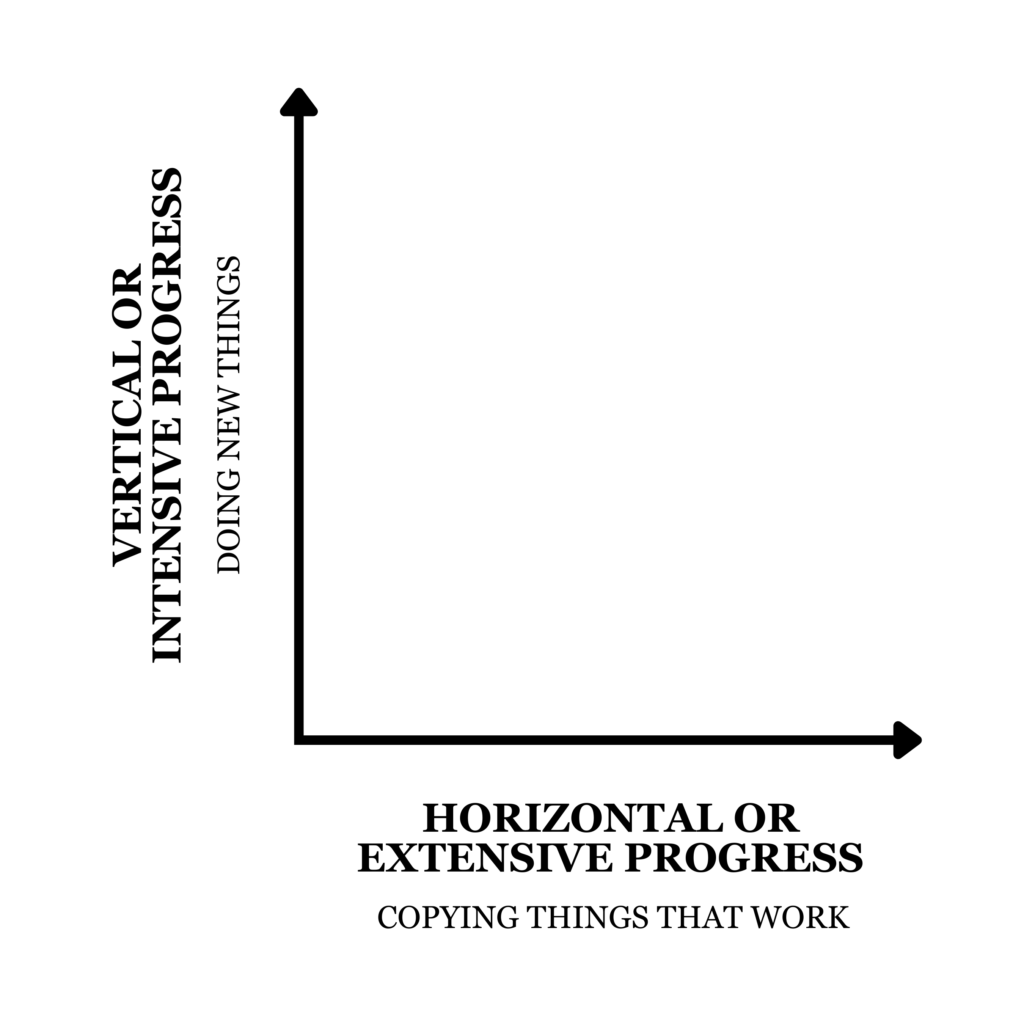
Thiel goes on to further define horizontal and vertical progress.
At the macro level, the single word for horizontal progress is globalization—taking things that work somewhere and making them work everywhere. . . . The single best word for vertical, 0 to 1 progress is technology. . . . Because globalization and technology are different modes of progress, it’s possible to have both, either, or neither at the same time.
Thiel’s Answer to the Contrarian Question
My own answer to the contrarian question is that most people think the future of the world will be defined by globalization, but the truth is that technology matters more. Without technological change, if China doubles its energy production over the next two decades, it will also double its air pollution. If every one of India’s hundreds of millions of households were to live the way Americans already do—using only today’s tools—the result would be environmentally catastrophic. Spreading old ways to create wealth around the world will result in devastation, not riches. In a world of scarce resources, globalization without new technology is unsustainable.
Conclusion
The point of the contrarian question is to glean new, uncommon perspectives. Thiel calls these secrets. All great ideas were once secrets—only a small number of people knew them. Their truth, once released to the world, became ubiquitous. Although beneficial for society as a whole, ubiquity doesn’t give you an edge; everyone knows the same thing.
Every one of today’s most famous and familiar ideas was once unknown and unsuspected. The mathematical relationship between a triangle’s sides, for example, was secret for millennia. Pythagoras had to think hard to discover it. If you wanted in on Pythagoras’s new discovery, joining his strange vegetarian cult was the best way to learn about it. Today, his geometry has become a convention—a simple truth we teach to grade schoolers. A conventional truth can be important—it’s essential to learn elementary mathematics, for example—but it won’t give you an edge. It’s not a secret.
Thiel’s contrarian question has the ability to spark fascinating conversation by unearthing secrets. And it can identify courageous, independent thinkers. That’s the beauty of well-designed questions: they deepen our understanding of the people and world around us.
What other questions might there be that will open new parts of your mind? What questions forge new, strong relationships? Collect questions like these and the depth and quality of your conversations will improve drastically. And the next time you’re in an interview, searching for more understanding of the person sitting across from you, your map will have much more detail of the territory because of inquiries like the contrarian question.